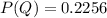Complete question
The complete question is shown on the first uploaded image
Answer:
a

b
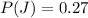
c

d
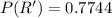
e
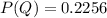
Explanation:
From the question we are told that
The proportion of M & M's that is brown is b = 0.12
The proportion of M & M's that is yellow is y = 0.15
The proportion of M & M's that is red is r = 0.12
The proportion of M & M's that is blue is b = 0.23
The proportion of M & M's that is orange is o = 0.23
The proportion of M & M's that is green is g = 0.15
Generally the probability that a random selected M&M is not yellow is

=>
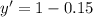
=>

Generally the probability that a randomly selected peanut M&M isgreen or red is mathematically represented as
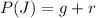
=>
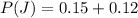
=>
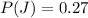
Generally the probability that two randomly selected peanut M&M’s are both red is mathematically represented as
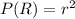
=>
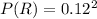
=>

Generally if two two peanut M&M’s are selected the probability that neither of them are red is mathematically represented as
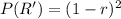
=>
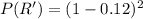
=>
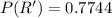
Generally if two two peanut M&M’s are selected the probability that at least one of them is red is mathematically represented as
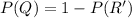
=>
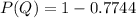
=>