Question

In ΔOPQ, p = 180 inches, q = 120 inches and ∠O=171°. Find the length of o, to the nearest inch.

Answer 1

Answer:299

Explanation:

Answer 2

Given:

In ΔOPQ, p = 180 inches, q = 120 inches and ∠O=171°.

To find:

The length of o, to the nearest inch.

Solution:

According to cosine formula,

`a^2=b^2+c^2-2bc\cos A`

Using cosine formula in ΔOPQ, we get

`o^2=p^2+q^2-2pq\cos O`

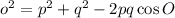
On substituting the values, we get

`o^2=(180)^2+(120)^2-2(180)(120)\cos (171^\circ)`

`o^2=32400+14400-43200(-0.9877)`

`o^2=46800+42668.64`

`o^2=89468.64`

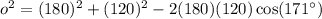
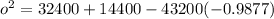
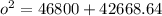
Taking square root on both sides.

`o=√(89468.64)`

`o=299.113`

`o\approx 299`

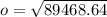
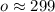
Therefore, the length of o is about 299 inches.