Answer:
1154
Explanation:
Perhaps the easiest way to evaluate this numerical expression is to let a calculator do it. Alternatively, we can compute the value from a +1/a.
__
expression
Consider the square of a +1/a:
(a +1/a)^2 = a^2 +2(a)(1/a) +1/a^2 = (a^2 +1/a^2) +2
This means ...
a^2 +1/a^2 = (a +1/a)^2 -2
Similarly, using a^2 for 'a' in the above, we have ...
a^4 +1/a^4 = (a^2 +1/a^2)^2 -2
numerical value
The value of a +1/a is ...
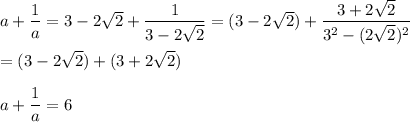
Then the value of a^2 +1/a^2 is 6^2 -2 = 34
and the value of a^4 +1/a^4 is 34^2 -2 = 1154