Answer:
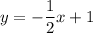
Explanation:
The equation of any line in slope-intercept form is:
y=mx+b
Being m the slope and b the y-intercept.
Assume we know the line passes through points A(x1,y1) and B(x2,y2). The slope can be calculated with the equation:
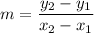
Two points are given: (-6,4) and (-2,2). Calculating the slope:

The equation of the line is, so far:
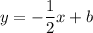
To calculate the value of b, we use any of the given points, for example (-6,4):
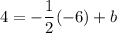
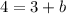
Solving:
b = 1
The equation of the line is:

We can see none of the choices is correct.