Answer:
The length of the legs of the triangle is approximately 86.85 meters
Explanation:
The requirement is to minimize the combined area of the two enclosures
The given parameters are;
The length of (perimeter) fencing available = 500 meters
The shape of the first enclosure = Square
The shape of the second enclosure = Triangle
The shape of the triangle = Isosceles right triangle
The area of the square = Side, S, squared = s²
The perimeter of the square = 4 × s
The area of the triangle = 1/2 × Base × Height = 1/2 × b × b= 1/2·b²
Where;
b = The lengths of the legs of the triangle
Given that the perimeter is 500 m, we have;
length of hypotenuse of triangle = √(b² + b²) =√(2·b²) = b·√2
∴ 500 - 4·s = b + b + b·√2 = 2·b + (√2)·b = b·(2 + √2)
b = (500 - 4·s)/(2 + √2)
∴ The area of the triangle = 1/2 × ((500 - 4·s)/(2 + √2))²
The area of the square + The area of the triangle = s² + 1/2 × ((500 - 4·s)/(2 + √2))²
At minimum value, we have;
d(s² + 1/2 × ((500 - 4·s)/(2 + √2))²/ds = 0, gives (Using online application)
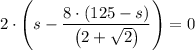
From which we have, s = 500/(7 + 2·√2)
Differentiating again gives;

Therefore, the point is a local minimum and the length of the legs of the triangle should be given as follows;
The length of the legs of the triangle = b = (500 - 4·s)/(2 + √2) = (500 - 4*(500/(7 + 2*√2)))/(2 + √2) ≈ 86.85
The length of the legs of the triangle ≈ 86.85 meters.