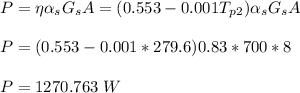Answer:
(a) the electrical power generated for still summer day is 1013.032 W
(b)the electrical power generated for a breezy winter day is 1270.763 W
Step-by-step explanation:
Given;
Area of panel = 2 m × 4 m, = 8m²
solar flux GS = 700 W/m²
absorptivity of the panel, αS = 0.83
efficiency of conversion, η = P/αSGSA = 0.553 − 0.001 K⁻¹ Tp
panel emissivity , ε = 0.90
Apply energy balance equation to determine he electrical power generated;
transferred energy + generated energy = 0
(radiation + convection) + generated energy = 0
![[\alpha_sG_s-\epsilon \alpha(T_p^4-T_s^4)]-h(T_p-T_\infty) - \eta \alpha_s G_s = 0](https://img.qammunity.org/2021/formulas/physics/college/m0q7lrnt4cx5hwbapkzkxnzt1r6dvygrae.png)
![[\alpha_sG_s-\epsilon \alpha(T_p^4-T_s^4)]-h(T_p-T_\infty) - (0.553-0.001T_p)\alpha_s G_s](https://img.qammunity.org/2021/formulas/physics/college/jjbq2mo21b6duuw5l32j8i3i3brvg9xk51.png)
(a) the electrical power generated for still summer day
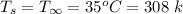
![[0.83*700-0.9*5.67*10^(-8)(T_p_1^4-308^4)]-10(T_p_1-308) - (0.553-0.001T_p_1)0.83*700 = 0\\\\3798.94-5.103*10^(-8)T_p_1^4 - 9.419T_p_1 = 0\\\\Apply \ \ iteration \ method \ to \ solve \ for \ T_p_1\\\\T_p_1 = 335.05 \ k](https://img.qammunity.org/2021/formulas/physics/college/447lwj0gthgltp5kgejppdlbuda624n4fs.png)
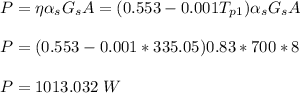
(b)the electrical power generated for a breezy winter day
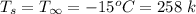
![[0.83*700-0.9*5.67*10^(-8)(T_p_2^4-258^4)]-10(T_p_2-258) - (0.553-0.001T_p_2)0.83*700 = 0\\\\8225.81-5.103*10^(-8)T_p_2^4 - 29.419T_p_2 = 0\\\\Apply \ \ iteration \ method \ to \ solve \ for \ T_p_2\\\\T_p_2 = 279.6 \ k](https://img.qammunity.org/2021/formulas/physics/college/5dnmnxl0wu0t0txr74uw8sgniypf7mov9p.png)