Question

Find the indicated conditional probability using the following two-way table

P(Take the bus|Junior)=[?]
round to the nearest hundredth.

Answer 1

P(Take the bus|Junior)=0.57

Explanation:

Conditional Probability

Is a measure of the probability of the occurrence of an event, given that another event has already occurred. If event B has occurred, then the probability that event A occurs is given by:

`{\displaystyle P(A\mid B)={\frac {P(A\cap B)}{P(B)}}}`

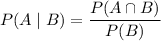
Where
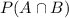`P(A\cap B)` is the probability that both events occur and P(B) is the probability that B occurs.

The two-way table shows statistics of students and we are interested to find the probability that, given the student is a junior (let's call it event B), they also take the bus (Event A). Thus, the probability we need to calculate is:

`\displaystyle P(A\mid B)={\frac {P(A\cap B)}{P(B)}}`

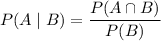
Checking on the table, we can see that both events occur when the row and the column of both events coincide, i.e. 20 students out of a total of 100 students in total. Thus:

`\displaystyle P(A\cap B)=\frac {20}{100}=0.2`

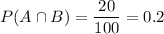
The probability that a student is classified as Junior is

`\displaystyle P(B)=\frac {35}{100}=0.35`

The conditional probability is:

`\displaystyle P(A\mid B)=\frac {0.2}{0.35}=0.5714`

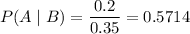
P(Take the bus|Junior)=0.57