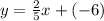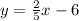Answer:
a.
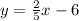
Explanation:
The slope-intercept form of equation of a line is given as
 . Where,
. Where,
m = slope
b = y-intercept.
Rewrite the equations,
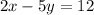 and
and
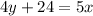 , in the slope-intercept form by making y the subject of the formula. Then, derive our new equation that has the same slope as the first equation, and the same y-intercept as the second equation.
, in the slope-intercept form by making y the subject of the formula. Then, derive our new equation that has the same slope as the first equation, and the same y-intercept as the second equation.
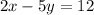
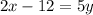
Divide both sides by 5


Rewrite

The slope of
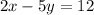 is ⅖.
is ⅖.
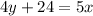
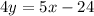 (subtraction property of equality)
(subtraction property of equality)
Divide both sides by 4
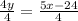
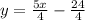
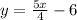
The y-intercet of
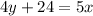 is -6
is -6
Therefore, the equation that has the same slope as the first equation and the same y-intercept as the second equation would be:

Plug in the values of m and b