Question

30 POINTS!!!!! (answer with work please)

1) A cone with volume 1350 m³ is dilated by a scale factor of 1/3. What is the volume of the resulting cone?

2) The top of the silo is a hemisphere with a radius of 11 ft. The bottom of the silo is a cylinder with a height of 38 ft. How many cubic feet of grain can the silo hold? Use 3.14 for pi and round your answer to the nearest cubic foot.

Answer 1

Answer: The answer is 50 m³.

Explanation: We are given to find the volume of the cone cone after being dilated by a factor of one-third from a cone with volume 1350 m³.

The volume of a cone with base radius 'r' units and height 'h' units is given by

`V=(1)/(3)\pi r^2h.`

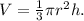
Therefore, if 'r' is the radius of the base of original cone and 'h' is the height, then we can write

`V=(1)/(3)\pi r^2h=1350`

⇒
`\pi r^2h=4050.`

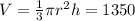
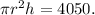
Now, if we dilate the cone by a scale factor of , then the radius and height will become one-third of the original one.

Therefore, the volume of the dilated cone will be

`V_d=(1)/(3)\pi ((r)/(3))^2(h)/(3) =(1)/(81)` ×
`\pi r^2h=(1)/(81)` ×
`4050=50`

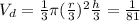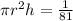Thus, the volume of the resulting cone will be 50 m³.