This is a multiplicative principle's question. Let's think how we can solve that.
If we want numbers are greater than 1000, so we need numbers with 4 digit (for example: 1001, 2350 and so on).
Imagine that we need to complete four spaces: one thousands, hundreds, tens and ones. See below how to compelete each space:
- One thousands: In this space, we can't use the number 0, because in this way, the number will have 3 digits and we want number with 4 digits (for example: 0235 is a 3 digits number). Therefore, we can use the numbers 2, 3 and 5 to complete this space. So, there are 3 possibilities.
- Hundreds: Now, we can use the number 0 and the number won't have 3 digits (for example: 2035 is a 4 digits number). Therefore, we can use the numbers 0, 2, 3 and 5 to complete this space. So, there are 4 possibililities.
- Tens: Ih this case, it happens the same thing that happen in the case of hundreds. Therefore, there are 4 possibilites.
- Ones: Finally, in this case it also happens the same thing that happen in the case os hundreds. So, there are 4 possibilites.
For multiplicative principle, we just need multiply these numbers of possibilities to find the result of this question:
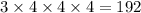
Therefore, we can write 192 numbers using the digits (0, 2, 3 and 5) and all these numbers are greater than 1000.