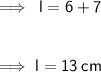Answer:
Let the width of the rectangle be w.
Let the length of the rectangle be l.
★ If the length is 3 feet longer than the width, then the length can be written in terms of the width as:
Now, the formula for finding the perimeter is p = 2l + 2w.
Substituting the values in this formula -
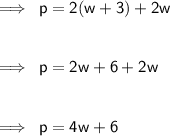
Substituting P = 30 for p ( allows us to solve for p) -
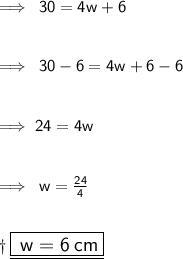
Substituting w = 6 for l in the equation l = w + 7 -