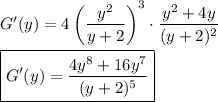Question

[Calc 1] An explanation on how to best solve this. I've been stuck trying to use chain rule

Answer 1

G'(y) = 4y^7(y+4)/(y+2)^5

Explanation:

Work it a piece at a time.

Define z = y^2/(y+2). Then your derivative is found from the power rule as ...

G = z^4

G' = 4z^3·z'

Now, define z = u/v. Then your derivative is found using the quotient rule:

z' = (vu' -uv')/v^2 = ((y+2)(2y) -(y^2)(1))/(y+2)^2

Putting this together we have ...

`G'(y)=4\left((y^2)/(y+2)\right)^3\cdot(y^2+4y)/((y+2)^2)\\\\\boxed{G'(y)=(4y^8+16y^7)/((y+2)^5)}`