Question

If 2y^2+2=x^2, then find d^2y/dx^2 at the point (-2, -1) in simplest form.​

Answer 1

`(d^2y)/(dx^2)_((-2, -1))=(1)/(2)`

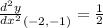
Explanation:

We have the equation:

`2y^2+2=x^2`

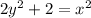
And we want to find d²y/dx² at the point (-2, -1).

So, let's take the derivative of both sides with respect to x:

`(d)/(dx)[2y^2+2]=(d)/(dx)[x^2]`

On the left, let's implicitly differentiate:

`4y(dy)/(dx)=(d)/(dx)[x^2]`

Differentiate normally on the left:

`4y(dy)/(dx)=2x`

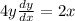
Solve for the first derivative. Divide both sides by 4y:

`(dy)/(dx)=(x)/(2y)`

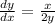
Now, let's take the derivative of both sides again:

`(d)/(dx)[(dy)/(dx)]=(d)/(dx)[(x)/(2y)]`

We will need to use the quotient rule:

`(d)/(dx)[f/g]=(f'g-fg')/(g^2)`

So:

`(d^2y)/(dx^2)=((d)/(dx)[(x)](2y)-x(d)/(dx)[(2y)])/((2y)^2)`

Differentiate:

`(d^2y)/(dx^2)=((1)(2y)-x(2(dy)/(dx)))/(4y^2)`

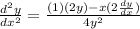
Simplify:

`(d^2y)/(dx^2)=(2y-2x(dy)/(dx))/(4y^2)`

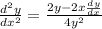
Substitute x/2y for dy/dx. This yields:

`(d^2y)/(dx^2)=(2y-2x(x)/(2y))/(4y^2)`

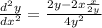
Simplify:

`(d^2y)/(dx^2)=(2y-(2x^2)/(2y))/(4y^2)`

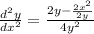
Simplify. Multiply both the numerator and denominator by 2y. So:

`(d^2y)/(dx^2)=(4y^2-2x^2)/(8y^3)`

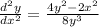
Reduce. Therefore, our second derivative is:

`(d^2y)/(dx^2)=(2y^2-x^2)/(4y^3)`

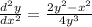
We want to find the second derivative at the point (-2, -1).

So, let's substitute -2 for x and -1 for y. This yields:

`(d^2y)/(dx^2)_((-2, -1))=(2(-1)^2-(-2)^2)/(4(-1)^3)`

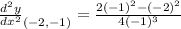
Evaluate:

`(d^2y)/(dx^2)_((-2, -1))=(2(1)-(4))/(4(-1))`

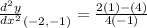
Multiply:

`(d^2y)/(dx^2)_((-2, -1))=(2-4)/(-4)`

Subtract:

`(d^2y)/(dx^2)_((-2, -1))=(-2)/(-4)`

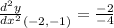
Reduce. So, our answer is:

`(d^2y)/(dx^2)_((-2, -1))=(1)/(2)`

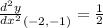
And we're done!