Answer:
CD ≈ 26.0 cm
Explanation:
using the sine ratio in right triangle ABD
sin35° =
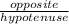 =
=
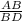 =
=
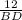 ( multiply both sides by BD )
( multiply both sides by BD )
BD × sin35° = 12 ( divide both sides by sin35° )
BD =
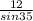 ≈ 20.92 cm
≈ 20.92 cm
using the sine rule in Δ BCD
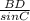 =
=
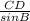 , that is
, that is
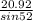 =
=
 ( cross- multiply )
( cross- multiply )
CD × sin52° = 20.92 × sin102° ( divide both sides by sin52° )
CD =
 ≈ 26.0 cm ( to 3 significant figures )
≈ 26.0 cm ( to 3 significant figures )