Answer:
1) p=1/2
2) V(p)=25
Explanation:
We have the function:
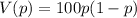
Which represents the variance of the number of left handed people in a group of 100.
Question 1)
We want to find the value of p that maximizes the variance.
Let's examine our function. We can see that it is a quadratic. Therefore, the value of p that maximizes the variance will simply be the x-coordinate of the vertex of our equation.
Let's expand our function:
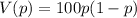
Distribute:
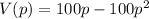
Let's find the vertex of our equation. We can use the following formulas (I switched out the x for p):

Let's determine our coefficients. The "a" is the coefficient in front of the squared term and "b" is the coefficient in front of the x term.
So, a is -100, and b is 100.
Substitute them into our formula:
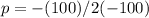
Multiply:
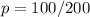
Divide:
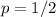
So, the x-coordinate (or rather p in this case) of our vertex is 1/2.
To find the y-value, let's substitute it back into our function. We have:
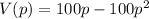
Substitute 1/2 for p:
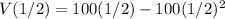
Evaluate:
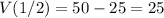
Therefore, our vertex is:
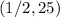
So, the value of p that maximizes our variance is p=1/2.
Question 2)
We want to find the maximum variance.
Again, this will simply be the vertex of our quadratic.
This time, it will be the y-coordinate.
We can see that the y-coordinate of the vertex is 25.
So, our maximum variance is V(p)=25.
And we're done!