Answer:
D
Explanation:
Recall that the area of a rectangle is given by the formula:

Where l is the the length and w is the width.
If we increase our length by 20%, this means that we add 20% or 0.2 of our old length to our old length. So, our new length will be:
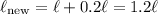
Similarly, if we increase our width by 10%, this means that we add 10% or 0.1 of our old width to our old width. So, our new width is:
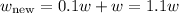
Therefore, our new area will be:
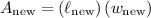
By substituting:
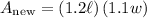
Multiply:
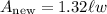
Recall that the old area is given by:
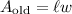
So, our area increased by a factor of 1.32. In other words, our area increased by 0.32 or 32%.
Therefore, our answer is D.