Question

Given that A’B’C’ is the image of ABC under a reflection, enter that equation of the line of reflection

Answer 1

The equation of the line of reflection is
`y = 2\cdot x +1`.

Explanation:

After looking carefullt the figure, we notice that line of reflection passes through B and midpoints in line segments AA' and CC'. If we know that
`A(x, y) = (-1, 4)`,
`A'(x, y) = (3, 2)`,
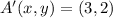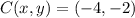`C(x, y) = (-4, -2)` and
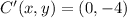`C'(x,y) = (0, -4)`, the midpoints of each line segment is:

Line segment AA'

`A'' = \left((-1+3)/(2),(4+2)/(2)\right)`

`A'' = (1,3)`

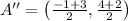
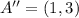
Line segment CC'

`C'' = \left((-4+0)/(2), (-2-4)/(2) \right)`

`C'' = (-2, -3)`

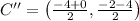
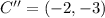
From Analytical Geometry we know that any linear function can be found by knowing two distinct points. The standard form of the linear function is represented by:

`y = m\cdot x+b`

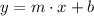
Where:

`x` - Independent variable, dimensionless.

`y` - Dependent variable, dimensionless.

`m` - Slope, dimensionless.

`b` - y-Intercept, dimensionless.

If we replace all variables with the components of the midpoints (
`A'' = (1,3)`,
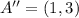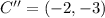`C'' = (-2, -3)`), then we get a system of two linear equations:

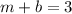`m + b = 3` (Eq. 1)

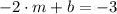`-2\cdot m + b = -3` (Eq. 2)

Lastly, we proceed to solve the system algebraically:

In (Eq. 1):

`b = 3-m`

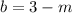
(Eq. 1) in (Eq. 2):

`-2\cdot m +3-m = -3`

`-3\cdot m + 3 = -3`

`-3\cdot m = -6`

`m = 2`

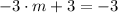
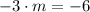
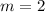
By (Eq. 1):

`b = 3-2`

`b = 1`

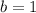
The equation of the line of reflection is
`y = 2\cdot x +1`.