Answer:
The 90% confidence interval

The null hypothesis is
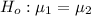
The alternative hypothesis
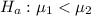
The distribution test statistics is

The rejection region is p-value <

The decision rule is reject the null hypothesis
The conclusion is
There is sufficient evidence to conclude that there are more passengers riding the 8:30 train
The p-value is
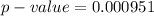
Step-by-step explanation:
From the question we are told that
The first sample size
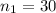
The first sample mean is
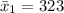
The first standard deviation is
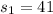
The second sample size is
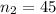
The second sample mean is
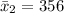
The second standard deviation is

given that the confidence level is 90% then the level of significance is mathematically represented as

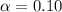
Generally the critical value of
 obtained from the normal distribution table is
obtained from the normal distribution table is

Generally the pooled variance is mathematically represented as
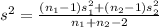
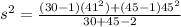

Generally the standard error is mathematically represented as

=>

=>

Generally the margin of error is mathematically evaluated as
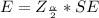

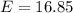
Generally the 90% confidence interval is mathematically represented as



The null hypothesis is
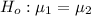
The alternative hypothesis
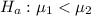
Generally the test statistics is mathematically represented as

=>
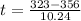
=>

Generally the degree of freedom is mathematically represented as
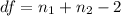

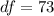
The p-value is obtained from the student t distribution table at degree of freedom of 73 at 0.05 level of significance
The value is
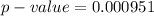
Here the level of significance is

Given that the p-value <
 then we reject the null hypothesis
then we reject the null hypothesis
Then the conclusion is
There is sufficient evidence to conclude that there are more passengers riding the 8:30 train