Answer:
The percentile rank for Park Street's revenues this week is 60th.
The percentile rank for Bridge Road's revenues this week is 73rd.
Explanation:
The missing information are as follows:
Variable N Mean SD
Park 36 6611 3580
Bridge 40 5989 1794
A z-score (aka, a standard score) specifies the number of standard deviations an observation is from the mean.
The formula to compute the z-score is,
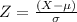 , where X = observation, µ = mean, σ = standard deviation.
, where X = observation, µ = mean, σ = standard deviation.
Compute the z-score for Park Street's revenues, $7500 as follows:
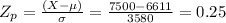
The z-score for Park Street's revenues this week is 0.25.
Compute the percentile rank for Park Street's revenues this week as follows:
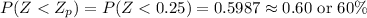
The percentile rank for Park Street's revenues this week is 60th.
This implies that the Park Street's performed better than 60% of the revenue recorded for the restaurant.
Compute the z-score for Bridge Road's revenues, $7100 as follows:
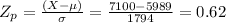
The z-score for Bridge Road's revenues this week is 0.62.
Compute the percentile rank for Bridge Road's revenues this week as follows:

The percentile rank for Bridge Road's revenues this week is 73rd.
This implies that the Bridge Road's performed better than 73% of the revenue recorded for the restaurant.