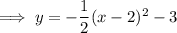Answer:
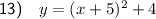
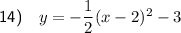
Explanation:
Vertex form of a parabola:
 where (h, k) is the vertex
where (h, k) is the vertex
Question 13
From inspection of the graph, the vertex is (-5, 4)
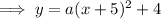
To find
 , substitute the coordinates of a point on the curve into the equation.
, substitute the coordinates of a point on the curve into the equation.
Using point (-4, 5):
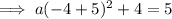


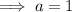
Therefore, the equation of the parabola in vertex form is:
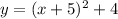
Question 14
From inspection of the graph, the vertex is (2, -3)
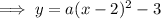
To find
 , substitute the coordinates of a point on the curve into the equation.
, substitute the coordinates of a point on the curve into the equation.
Using point (0, -5):
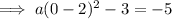
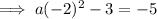



Therefore, the equation of the parabola in vertex form is: