Question

Please help. I need help with number 76.

Answer 1

Answer:
`12x^2+12xh+4h^2-2`

==================================================

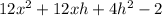
Step-by-step explanation:

First let's find the expression f(x+h). We replace every copy of x with x+h, expand, and simplify like so.

`f(x) = 4x^3 - 2x\\\\f(x+h) = 4(x+h)^3 - 2(x+h)\\\\f(x+h) = 4(x+h)(x+h)^2 - 2(x+h)\\\\f(x+h) = 4(x+h)(x^2+2xh+h^2) - 2(x+h)\\\\f(x+h) = 4x(x^2+2xh+h^2)+4h(x^2+2xh+h^2) - 2(x+h)\\\\f(x+h) = 4x^3+8x^2h+4xh^2+4hx^2+8xh^2+4h^3 - 2x-2h\\\\f(x+h) = 4x^3+12x^2h+12xh^2+4h^3 - 2x-2h\\\\`

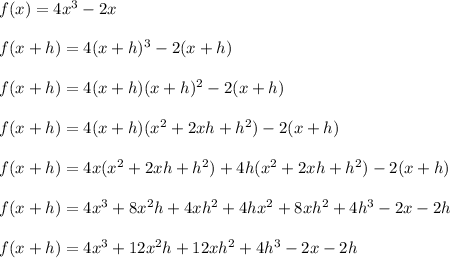
This may look ugly, but things will simplify a bit when we subtract off f(x)

Note how in f(x+h) we have the following terms

• 4x^3
• -2x

Which are the exact terms found in f(x) as well.

Why is this important? It helps us subtract and cancel. When we subtract off f(x), we cross off the 4x^3 and -2x terms in that f(x+h) equation above.

We'll be left with this

`f(x+h)-f(x) = 12x^2h+12xh^2+4h^3-2h\\\\`

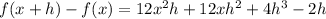
At this point, every term shown has at least one copy of 'h' as a factor.

This allows us to factor out h

`f(x+h)-f(x) = h(12x^2+12xh+4h^2-2)\\\\`

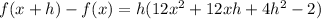
Then we can divide both sides by h to get our final answer

`(f(x+h)-f(x))/(h) = (h(12x^2+12xh+4h^2-2))/(h)\\\\(f(x+h)-f(x))/(h) = 12x^2+12xh+4h^2-2\\\\`

----------------------------------------------------

Extra info:

h is nonzero to avoid dividing by zero. Though as h gets closer and closer to zero, terms with h in them will drop out since those terms will be very small. We'll be left with 12x^2-2. This is the derivative of f(x).