Question

The compound 2-hydroxybiphenyl (o-phenylphenol) boils at 286 °C under 101.325 kPa and at 145 °C under a reduced pressure of

14 Torr. Calculate the value of the molar enthalpy of vaporization. Compare this value to that given in the CRC Handbook.​

Answer 1

Answer:
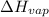`\Delta H_(vap)` = 55.1 kJ/mol

Explanation: Molar Enthalpy of Vaporization(
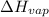`\Delta H_(vap)` ) is the energy needed to change 1 mol of a substance from liquid to gas at constant temperature and pressure.

For the 2-hydroxybiphenyl, there two temperatures and 2 pressures. In this case, use Clausius-Clapeyron equation:

`ln(P_(2))/(P_(1))=(\Delta H_(vap))/(R) ((1)/(T_(1))-(1)/(T_(2)) )`

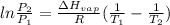
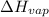`\Delta H_(vap)` is in J/mol:

1) Temperature in K

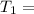`T_(1)=` 286 +273 = 559K

`T_(2)` = 145 + 273 = 418K

2) Both pressure in Pa

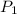`P_(1)` = 101325Pa

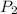`P_(2)` = 14*133 = 1862Pa

Since molar enthalpy is in Joules, gas constant R is 8.3145J/mol.K

Replacing into the equation:

`ln(1862)/(101325)}=(\Delta H_(vap))/(8.3145) ((1)/(559)-(1)/(418) )`

`ln(0.0184)=(\Delta H_(vap))/(8.3145) ((141)/(233662) )`

`\Delta H_(vap)=(-3.9954*1942782.7)/(-141)`

`\Delta H_(vap)=55051.02`

`\Delta H_(vap)=55051.02`

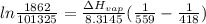
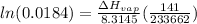
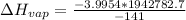
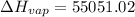
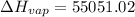
`\Delta H_(vap)=55.1` kJ/mol

Using those values, molar enthalpy is 55.1 kJ/mol

Comparing to the CRC Handbook, which is
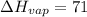`\Delta H_(vap)=71` kJ/mol:

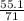`(55.1)/(71)` = 0.78

The calculated value is 0.78 times less than the CRC Handbook.