Question

Jane drew a point A located at (-3,-0) and point Clocated at (12,-6). What is the x-coordinate of point B that bisects AC?

Answer 1

The x-coordinate of point B that bisects AC is
`(9)/(2)`.

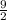Explanation:

The statement is not correct, the correct form is:

Jane drew a point A located at (-3,0) and point Clocated at (12,-6). What is the x-coordinate of point B that bisects AC?

Where a point bisects a segment, it means that segment is divided into two equal parts. If we know that
`\vec A = (-3, 0)` and
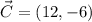`\vec C = (12, -6)` are endpoints of the segment, the location of the endpoint can be found by the following vectorial formula:

`\vec B = \vec A + (1)/(2)\cdot \overrightarrow {AC}`

`\vec B = \vec A + (1)/(2) \cdot (\vec C-\vec A)`

`\vec B = (1)/(2)\cdot \vec A + (1)/(2)\cdot \vec C`

`\vec B = (1)/(2)\cdot (-3,0)+(1)/(2)\cdot (12,-6)`

`\vec B = \left(-(3)/(2), 0 \right)+\left(6,-3\right)`

`\vec B = \left(-(3)/(2)+6, 0-3\right)`

`\vec B = \left((9)/(2),-3\right)`

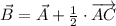
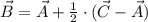
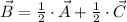
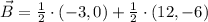
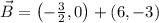
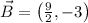
The x-coordinate of point B corresponds to the first component of the ordered pair found above, that is,
`x_(B) = (9)/(2)`.

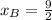The x-coordinate of point B that bisects AC is
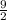`(9)/(2)`.