Question

Write an equation of a parabola that passes through (3,-30) and has x-intercepts of -2 and 18. Then find the average rate of change from x= -2 to x=8.

Answer 1

The equation of the parabola is
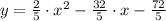`y = (2)/(5)\cdot x^(2)-(32)/(5)\cdot x -(72)/(5)`. The average rate of change of the parabola is -4.

Explanation:

We must remember that a parabola is represented by a quadratic function, which can be formed by knowing three different points. A quadratic function is standard form is represented by:

`y = a\cdot x^(2)+b\cdot x + c`

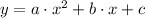
Where:

`x` - Independent variable, dimensionless.

`y` - Dependent variable, dimensionless.

`a`,
`b`,
`c` - Coefficients, dimensionless.

If we know that
`(3, -30)`,
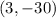`(-2, 0)` and
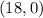`(18, 0)` are part of the parabola, the following linear system of equations is formed:

`9\cdot a +3\cdot b + c = -30`

`4\cdot a -2\cdot b +c = 0`

`324\cdot a +18\cdot b + c = 0`

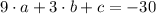
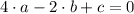
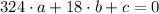
This system can be solved both by algebraic means (substitution, elimination, equalization, determinant) and by numerical methods. The solution of the linear system is:

`a = (2)/(5)`,
`b = -(32)/(5)`,
`c = -(72)/(5)`.

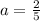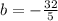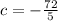The equation of the parabola is
`y = (2)/(5)\cdot x^(2)-(32)/(5)\cdot x -(72)/(5)`.

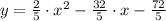Now, we calculate the average rate of change (
`r`), dimensionless, between
`x = -2` and
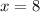`x = 8` by using the formula of secant line slope:

`r = (y(8)-y(-2))/(8-(-2))`

`r = (y(8)-y(-2))/(10)`

`x = -2`

`y = (2)/(5)\cdot (-2)^(2)-(32)/(5)\cdot (-2)-(72)/(5)`

`y(-2) = 0`

`x = 8`

`y = (2)/(5)\cdot (8)^(2)-(32)/(5)\cdot (8)-(72)/(5)`

`y(8) = -40`

`r = (-40-0)/(10)`

`r = -4`

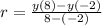
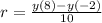
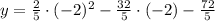
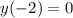
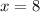
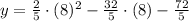
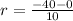
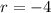
The average rate of change of the parabola is -4.