Question

Find the cardinal number of the set
C = x € N, 30 < x < 56 and x is divisible by 6,

Answer 1

Answer: 4

=====================================================

Step-by-step explanation:

We're given the following

`C = \{ x | x \in \mathbb{N}, \ 30 < x < 56, \ \text{ x is divisible by 6}\}`

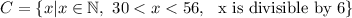
which means that we have a set of natural numbers between 30 and 56, excluding both endpoints, and the set of numbers are also multiples of 6.

So looking at the multiples of 6 that are between 30 and 56 leads us to

{30, 36, 42, 48, 54}

but we cross off 30 as we want x to be larger than that, so we get this updated final list

{36, 42, 48, 54}

There are four items in this final list so the cardinality of the set described is 4. The cardinality is just the number of items in a given set.

Answer 2

The cardinal number of set C is 4, representing the count of natural numbers between 30 and 56 that are divisible by 6.

Step-by-step explanation:

To find the cardinal number of set C = x ∈ N, 30 < x < 56 and x is divisible by 6, we need to count the natural numbers greater than 30 and less than 56 that are divisible by 6. These numbers are 36, 42, 48, and 54. Therefore, the cardinal number of set C is 4, as there are four numbers in the set that meet the given criteria.