Question

At the instant a traffic light turns green, a car that has been waiting at the intersection starts moving with a constant acceleration of 2.5 m/s/s. At that moment a truck traveling with a constant velocity of 15.0 m/s is at the same position along the road and passes the car in the next lane. Calculate the distance beyond the traffic light where the car and the truck will be side-by-side.

Answer 1

d = 180 m.

Step-by-step explanation:

We can find the distance where the car and the truck will be side-by-side as follows:

For the car we have:

`X_(f) = X_(0) + V_(0)t + (1)/(2)a_(c)t^(2)`

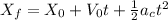
Where:

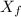`X_(f)` is the final position

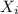`X_(i)` is the initial position = 0

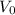`V_(0)` is the initial speed = 0

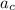`a_(c)`: is the acceleration = 2.5 m/s²

t: is the time

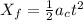`X_(f) = (1)/(2)a_(c)t^(2)` (1)

For the truck we have:

`X_(f) = X_(0) + V_(0)t + (1)/(2)at^(2)`

`X_(f) = 0 + V_(0)t + (1)/(2)0*t^(2)`

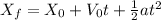
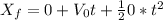
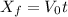`X_(f) = V_(0)t` (2)

By equating equation (1) and (2) we have:

`(1)/(2)a_(c)t^(2) = V_(0)t`

`t = (2V_(0))/(a_(c)) = (2*15 m/s)/(2.5 m/s^(2)) = 12 s`

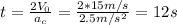
Since the car and the truck will be side-by-side at 12 seconds, we can calculate now the distance:

`X_(f) = V_(0)t = 15 m/s*12 s = 180 m`

Therefore, the distance at which the car and the truck will be side-by-side is 180 m.

I hope it helps you!