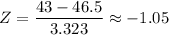Question

A sample of 10 packs of a brand of chewing gum was

taken. Each pack was weighed and their weights, in
grams, are shown.
What is the Z-score for the pack of gum weighing 43
grams?
01.13
43.0, 43.7, 49.6, 46.9, 47.6, 45.4, 51.2, 48.0, 40.5, 49.1
11.05
01.05
01.13

Answer 1

The z-score is approximately -1.05

Explanation:

The given data of the weights of the packs of chewing gum are;

43.0, 43.7, 49.6, 46.9, 47.6, 45.4, 51.2, 48.0, 40.5, and 49.1

The number of chewing gums in the sample, n = 10

The sum of the weights of the chewing gums is therefore;

43.0+43.7+49.6+46.9+47.6+45.4+51.2+48.0+40.5+49.1 = 465

The average weight is given as follows;

The average weight of the chewing gums = (The sum of the weights of the chewing gums)/(The number chewing gums)

The average weight, μ, of the chewing gums = (465)/(10) = 46.5

The standard deviation, σ, is given by the formula;

`\sigma =\sqrt{(\sum (x_(i) - \mu )^(2))/(n-1)}`

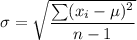
Where;

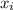`x_i` = Each individual chewing gum weight values

With the standard deviation formula in Excel, we have;

σ ≈ 3.323 grams

The z-score, z, is given by the following formula;

`Z=(x-\mu )/(\sigma )`

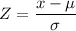
Therefore, the z-score of 43 is given as follows;

`Z=(43-46.5 )/(3.323 ) \approx -1.05`