Answer:
34,220
Explanation:
Because order doesn't matter, but the numbers can't be repeated, we need to find the number of combinations where 3 individual numbers can be chosen out of 60 possible numbers using the binomial coefficient:
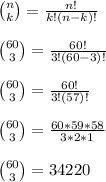
Thus, Elias can make 34,220 unique 3-number codes given 60 different numbers.