Hey ! there
Answer:
- Length of rectangle is 8 cm .
Explanation:
In this question we are provided with a rectangle having area 48 cm² and width 6 cm . We are asked to find the length of rectangle .
We know that ,
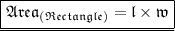
Where ,
SOLUTION : -
We are finding value of length by substituting value of width as 6 cm and area as 48 cm² in the formula . So ,

or ,

Dividing with 6 on both sides :
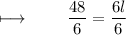
We get ,
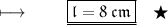
- Henceforth , Length of rectangle having area 48 cm² and width 6 cm is ❝ 8 cm ❞
Verifying : -
We are verifying our answer by substituting value of length and width in formula and equating it with given area . So ,
Therefore , our answer is correct .
#Keep Learning