Question

At a certain location, wind is blowing steadily at 10 m/s. Determine the mechanical energy of air per unit mass and the power generation potential of a wind turbine with 70-m-diameter blades at that location. Also determine the actual electric power generation assuming an overall efficiency of the wind turbine to be 30 percent. Take the air density to be 1.25 kg/m^3.

Answer 1

a) 50 J/kg

b) 721 67 KW

Step-by-step explanation:

The velocity of the wind v = 10 m/s

diameter of the blades d = 70 m

efficiency of the turbine η = 30%

density of air ρ = 1.25 kg/m^3

The area of the blade A =
`\pi d^2/4`

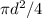
A =
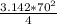`(3.142 * 70^2)/(4)` = 3848.95 m^2

The mechanical energy air per unit mass is gives as

e =
`v^2/2` =
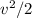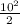`(10^2)/(2)` = 50 J/kg

Theoretical Power of the turbine P = ρAve

where

ρ is the density of air

A is the area of the blade

v is the velocity of the wind

e is the energy per unit mass

substituting values, we have

P = 1.25 x 3848.95 x 10 x 50 = 2405593.75 W

Actual power = ηP

where η is the efficiency of the turbine

P is the theoretical power of the turbine

Actual power = 0.3 x 2405593.75 = 721678.1 W

==> 721 67 KW