Question

Alcohol consumption slows people's reaction times. In a controlled government test, it takes a certain driver t1=0.27 st1=0.27 s to hit the brakes in a crisis when unimpaired and t2=1.05 st2=1.05 s when drunk. If the car is initially traveling at v=89 km/hv=89 km/h , how much farther does the car travel before the brakes are applied when the person is drunk than it travels when the person is sober?

Answer 1

The car travels 19.28 m further before the brakes are applied

Step-by-step explanation:

To determine how much farther the car travels before the brakes are applied when the person is drunk than it travels when the person is sober,

We will determine the distance covered when the driver is drunk and the distance covered when the driver is sober, the difference in the distances gives how much farther the car travels.

Let the distance covered when the person is unimpaired be
`x_(1)`

and the distance when the person is drunk be
`x_(2)`

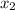
From the question,

The time it takes a certain driver to hit the brakes in a crisis when unimpaired is

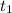`t_(1)` = 0.27 s

and the time it takes a certain driver to hit the brakes in a crisis when drunk is

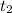`t_(2)` = 1.05 s

Also, from the question,

The car is traveling as 89 km/h

First, convert this to m/s

(NOTE: 1km = 1000m and 1h = 3600s)

Then,

89 km/h = 89 × 1000m / 3600s

= 24.72 m/s

This is the velocity of the car

Now, from

Velocity = Change in distance / Change in time

That is,

`Velocity = ( \Delta x )/( \Delta t )`

`Velocity = (x_(2) - x_(1) )/(t_(2) - t_(1) )`

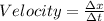
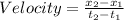
Then,

`24.72 = (x_(2) - x_(1) )/(1.05 - 0.27 )`

`24.72 = (x_(2) - x_(1) )/(0.78 )\\`

`{x_(2) - x_(1) } = 24.72 * 0.78`

`{x_(2) - x_(1) } = 19.28m`

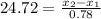
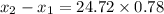
This is the difference in the distances covered when the driver is drunk and when the driver is sober.

Hence, the car travels 19.28 m further before the brakes are applied