Question

olecular iodine, I2(g), dissociates into iodine atoms at 625 K with a first-order rate constant of 0.271 s-1. (a) What is the half-life for this reaction? s (b) If you start with 0.048 M I2 at this temperature, how much will remain after 5.37 s assuming that the iodine atoms do not recombine to form I2? M

Answer 1

*
`t_(1/2)=2.56s`

*
`[I_2]=0.011M`

Step-by-step explanation:

Hello,

In this case, considering the reaction:

`I_2(g)\rightarrow 2I`

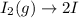
Which is first-order with respect to I₂, we can compute the half-life by:

`t_(1/2)=(ln(2))/(k)=(ln(2))/(0.271s^(-1))\\ \\t_(1/2)=2.56s`

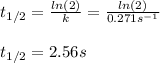
Moreover, since the integrated rate law is:

`[I_2]=[I_2]_0exp(-kt)`

We can compute the concentration of iodine once 5.37 s have passed:

`[I_2]=0.048Mexp(-0.271s^(-1)*5.37s)\\\\`

`[I_2]=0.011M`

Best regards.