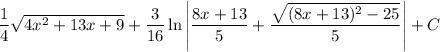Integral of x+2/ \sqrt{x { + 2x + 3}^(2) }

Question

asked May 10, 2021 39.2k views

1 Answer

Mario Corchero · Answer 1 · 2021-05-16T14:55:36+0000

Looks like the integral should be

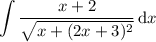
$\displaystyle\int(x+2)/(√(x+(2x+3)^2))\,\mathrm dx$

Expand the denominator:

$\displaystyle\int(x+2)/(√(4x^2+13x+9))\,\mathrm dx$

Notice that

$u=4x^2+13x+9\implies \mathrm du=(8x+13)\,\mathrm dx$

In order to use this substitution, expand the integral as

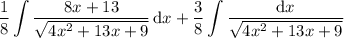
$\displaystyle\frac18\int(8x+13)/(√(4x^2+13x+9))\,\mathrm dx+\frac38\int(\mathrm dx)/(√(4x^2+13x+9))$

In the first integral, use the previously mentioned substitution:

$\displaystyle\frac18\int(8x+13)/(√(4x^2+13x+9))\,\mathrm dx=\frac18\int(\mathrm du)/(\sqrt u)=\frac14\sqrt u$

$=\frac14√(4x^2+13x+9)$

In the second integral, complete the square in the denominator:

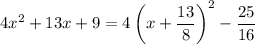
$4x^2+13x+9=4\left(x+\frac{13}8\right)^2-(25)/(16)$

Now substitute

$x=\frac58\sec t-\frac{13}8\implies\mathrm dx=\frac58\sec t\tan t\,\mathrm dt$

so that

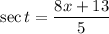
$\sec t=\frac{8x+13}5$

Then

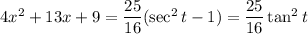
$4x^2+13x+9=(25)/(16)(\sec^2t-1)=(25)/(16)\tan^2t$

and the integral becomes

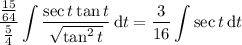
$\displaystyle((15)/(64))/(\frac54)\int(\sec t\tan t)/(√(\tan^2t))\,\mathrm dt=\frac3{16}\int\sec t\,\mathrm dt$

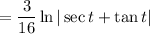
$=\frac3{16}\ln|\sec t+\tan t|$

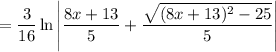
$=\frac3{16}\ln\left|\frac{8x+13}5+\frac{√((8x+13)^2-25)}5\right|$

So, the integral is

$\frac14√(4x^2+13x+9)+\frac3{16}\ln\left|\frac{8x+13}5+\frac{√((8x+13)^2-25)}5\right|+C$