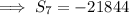Answer:
-21844
Explanation:
Given:
First find n by using the general form of a geometric sequence:
 (where a is the first term and r is the common ratio)
(where a is the first term and r is the common ratio)
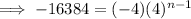
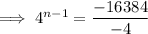
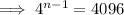
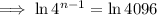
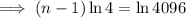
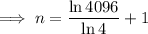
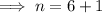
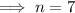
Sum of the first n terms of a geometric series:
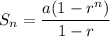
(where a is the first term and r is the common ratio)
Substituting the given values and the found value of n into the formula: