Answer:
Option A
Explanation:
Since the "y" variable is inside a root, we need to square both sides of the equation to open the root and solve for y.
When squaring both sides, we get;
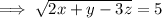

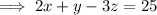
Now, simply isolate the y-variable to determine its value. This can be done by subtracting 2x on both sides of the equation.
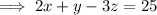
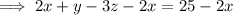
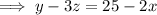
Now, add 3z both sides of the equation to further isolate the y-variable.
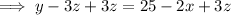
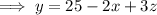
Therefore, Option A is correct.