Answer:
6 terms
Explanation:
we have
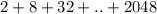
we need to identify a pattern so
from 2 to 8 what could have happened?
well you could have added 6 and get 8
so that means that to 8 you have to add 6 and get 32
WRONG
so adding is not an option
what else could have happened?
well you could have multiplied by 4 and get 8
so that means that to 8 you have to multiply by 4 and get 32
which is true
so the geometric series is
 what?
what?
you can see that in the 2nd term we have 1 four, in the 3rd we have 2 fours
so we can conclude in the nth term we have n-1 fours
so the formula is
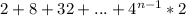
so we need to determine when

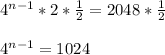
if we factorize 4 as
 and 1024 as
and 1024 as
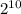
we have
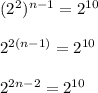
so we see that the bases are equal, that must mean the exponents are equal
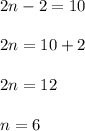
so there are 6 terms