Answer:
a = 0.161

Step-by-step explanation:
Given :
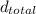 = 10 km = 10000 m
= 10 km = 10000 m
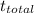 = 27 min 43.6 s
= 27 min 43.6 s
= 1663.6 s
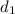 = 7.85 km = 7850 m
= 7.85 km = 7850 m
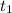 = 25 min = 1500 s
= 25 min = 1500 s
 = 60 s
= 60 s
Now the initial speed for the distance of 7.85 km is
 = 5.23 m/s
= 5.23 m/s
The velocity after 60 s after the distance of 7.85 kn is

= 5.23 + a(60)
The distance traveled for 60 s after the distance of 7.85 km is
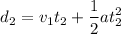
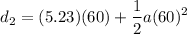
= 313.8 + a(1800)
The time taken for the last journey where the speed is again uniform is

= 1663.6 - 1500 - 60
= 103.6 s
Therefore, the distance traveled for the time
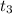 is
is
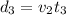
= (5.23+60a)(103.6)
= 541.8 + 6216 a
The total distance traveled,
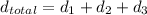
Now substituting the values in the above equation for the acceleration a is
10000 = 7850 + (313.6 + 1800a) + (541.8 + 6216a)
10000 = 8706.5 + 8016a
1294.4 = 8016a
a = 0.161
