Answer:
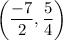 .
.
Explanation:
It is given that Point A is at (-5, -4) and point B at (-3, 3).
We need to find the coordinates of the point which is 3/4 of the way from A to B.
Let the required point be P.
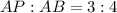
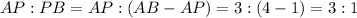
It means, point P divides segment AB in 3:1.
Section formula: If a point divides a line segment in m:n, then
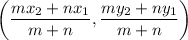
Using section formula, we get
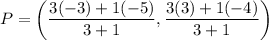

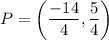

Therefore, the required point is
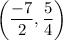 .
.