Answer:
a) The mean stress experimented by the aluminium part is 100 megapascals, b) The stress amplitude of the aluminium part is 400 megapascals, c) The stress ratio of the aluminium part is 4.
Step-by-step explanation:
a) The mean stress is determined by this expression:
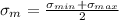
Where:
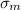 - Mean stress, measured in megapascals.
- Mean stress, measured in megapascals.
 - Minimum stress, measured in megapascals.
- Minimum stress, measured in megapascals.
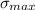 - Maximum stress, measured in megapascals.
- Maximum stress, measured in megapascals.
If we know that
 and
and
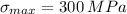 , the mean stress is:
, the mean stress is:
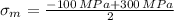

The mean stress experimented by the aluminium part is 100 megapascals.
b) The stress amplitude is given by the following difference:
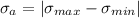
Where
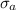 is the stress amplitude, measured in megapascals.
is the stress amplitude, measured in megapascals.
If we know that
 and
and
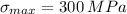 , the stress amplitude is:
, the stress amplitude is:
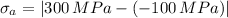
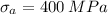
The stress amplitude of the aluminium part is 400 megapascals.
c) The stress ratio (
 ) is the ratio of the stress amplitude to mean stress. That is:
) is the ratio of the stress amplitude to mean stress. That is:
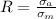
If we know that
 and
and
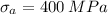 , the stress ratio is:
, the stress ratio is:

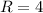
The stress ratio of the aluminium part is 4.