Question

You are given two vectors vector A = 4.9 at 31o vector B = 6 at 156o Angles are measured counterclockwise from the x-axis. What is the magnitude and direction of vector C = vector A + vector B? Magnitude = Direction = o

Answer 1

C_{y} = 4.96 and θ' = 104,5º

Step-by-step explanation:

To add several vectors we can decompose each one of them, perform the sum on each axis, to find the components of the resultant and then find the module and direction.

Let's start by decomposing the two vectors.

Vector A

sin θ =
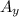`A_(y)` / A

cos θ = Aₓ / A

A_{y} = A sin θ

Ax = A cos θ

A_{y} = 4.9 sin 31 = 2.52

Ax = 4.9 cos 31 = 4.20

Vector B

B_{y} = B sin θ

Bx = B cos θ

B_{y} = 6 sin 156 = 2.44

Bx = 6 cos 156 = -5.48

The components of the resulting vector are

X axis

Cx = Ax + B x

Cx = 4.20 -5.48

Cx = -1.28

Axis y

C_{y} = Ay + By

C_{y} = 2.52 + 2.44

C_{y} = 4.96

Let's use the Pythagorean theorem to find modulo

C = √ (Cₙ²x2 + Cy2)

C = Ra (1.28 2 + 4.96 2)

C = 5.12

We use trigonemetry to find the angle

tan θ = C_{y} / Cₓ

θ’ = tan⁻¹ (4.96 / (1.28))

θ’ = 75.5

como el valor de Cy es positivo y Cx es negativo el angulo este en el segundo cuadrante, por lo cual el angulo medido respecto de eje x positivo es

θ’ = 180 – tes

θ‘= 180 – 75,5

θ' = 104,5º