2 Answers

Morten Holmgaard · Answer 1 · 2021-05-18T18:44:59+0000

In order for this polynomial (with respect to x) to have real roots, its discriminant should be non negative.

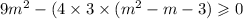
$9 {m}^(2) - (4 * 3 * ( {m}^(2) - m - 3) \geqslant 0$

Which means

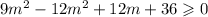
$9 {m}^(2) - 12 {m}^(2) + 12m + 36 \geqslant 0$

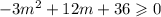
$- 3 {m}^(2) + 12m + 36 \geqslant 0$

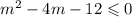
${m}^(2) - 4m - 12 \leqslant 0$

The last inequality is also a polynomial inequality.

The polynomial (with respect to m) has the following discriminant:

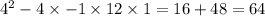
${4}^(2) - 4 * - 1 * 12 * 1 = 16 + 48 = 64$

Thus the roots of this polynomial are:

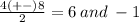
$(4( + - )8)/(2) = 6 \: and \: - 1$

When a polynomial has two positive roots named y, z for example where y<z the sign of the polynomial goes as following.

If the coefficient of the highest order term is positive then the polynomial is positive for m<y and m>z and negative for y<m<z.

If the coefficient of the highest order term is negative then the polynomial is negative for m<y and m>z and positive for y<m<z.

In the polynomial:

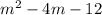
${m}^(2) - 4m - 12$

The coefficient of the highest order term is 1 thus the polynomial is non positive at the interval [-1,6].

Thus the solution to the exercise is

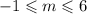
$- 1 \leqslant m \leqslant 6$

I apologize for any typos or wrong calculations.

Please log in or register to add a comment.