Answer:
AB is 31.
Explanation:
So we know that B is the midpoint of AC. This means that:
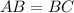
by the definition of midpoint.
We know that AB is 2x+1 and BC is 5x-44. Substitute:
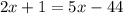
Now, find the value of x.
Subtract 1 from both sides:

Subtract 5x from both sides:
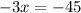
Divide both sides by -3:
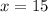
So, the value of x is 15.
AB is 2x+1.
Substitute 15 into x. So:

Multiply:

Add:

So, the length of AB is 31.
And we're done!