Question

The length of a rectangular floor is 7 feet longer than its width w. The area of the floor is 505 ft2

.
(a) Write a quadratic equation in terms of w that represents the situation.
(b) What are the dimensions of the floor? Round to the nearest tenth.
Show your work.

Answer 1

Width is 19.24 ft and length is 24.24 ft

Explanation:

Step 1: Write an equation

`A = l * w`

`505\ ft^2 = (7+w) * w`

`505\ ft^2 = (w*w) + (w*7)`

`505\ ft^2 - 505\ ft^2 = w^2 + 7w - 505\ ft^2`

`0= w^2 + 7w - 505\ ft^2`

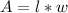
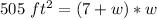
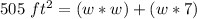
Step 2: Determine the dimensions

Use Quadratic formula

`x=(-b\pm√(b^2-4ac))/(2a)`

`x=(-7\pm√((7)^2-4(1)(-505)))/(2(1))`

`x=(-7\pm√(2069))/(2)`

`x = (-7\pm45.486)/(2)`

`x=19.24,\ -26.24`

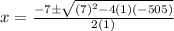
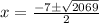
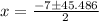
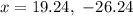
Since length cannot be negative, we will use 19.24 for x.

Step 3: Determine the length

`l = w + 5`

`l = 19.24 + 5`

`l = 24.24`

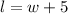
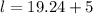
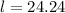
Answer: Width is 19.24 ft and length is 24.24 ft