Question

Here is the question, please answer

Answer 1

m∠5 = 118°

m∠7 = 118°

Explanation:

We have to note angle relationships here.

Since there are two parallel lines are being cut by a transversal, that means all the angles that are on one “angle plane” will be equal to the other.

This means that m∠2 = m∠6. Since we know m∠2 is 62°, that means m∠6 is also 62°.

We also know that m∠6 and m∠5 are supplementary, meaning their angles add up to 180°. Since we already know m∠6 we can find m∠5 easily.

`180 - 62 = 118`

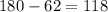
So m∠5 = 118°.

m∠5 and m∠7 are opposite angles, meaning that they will be the exact same. This also makes m∠7 118°.

Hope this helped!