Answer:
The radius of the volleyball is 8.3 inches
Explanation:
Given
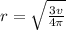
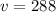
Required
Determine the value of r
To do this, we simply substitute 288 for v and 3.14 for π in the given equation.
This gives
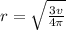
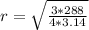
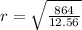
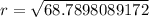
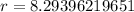
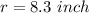 (Approximated)
(Approximated)
Hence;
The radius of the volleyball is 8.3 inches