Answer:
The equation
 represents in R^2 c) a line
represents in R^2 c) a line
The equation
 represents in R^3 a) a plane
represents in R^3 a) a plane
The equation
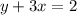 represents in R^3 a) a plane
represents in R^3 a) a plane
The equation
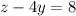 represents d) a plane
represents d) a plane
The pair of equations
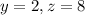 represents a) a line
represents a) a line
Explanation:
Let's start by studying each question :
1)
In R^2 ,
 with
with
 ∈ IR is the equation of all vertical lines
∈ IR is the equation of all vertical lines
In this case, the only free variable is the variable ''y''
R^2 has two dimensions (x and y) so if we set
 we will have only one free variable in R^2 (which is a line in R^2). Therefore,
we will have only one free variable in R^2 (which is a line in R^2). Therefore,
 represents a line in R^2
represents a line in R^2
Now, in R^3 we have three dimensions (x, y and z) so if we set
 we will have only two free variables (y and z) and
we will have only two free variables (y and z) and
 will represent a plane (which have two dimensions) in R^3.
will represent a plane (which have two dimensions) in R^3.
2) The equation
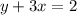 in R^3 has the free variable ''z'' and given that we select a value (for example for ''x'') the another value from the variable ''y'' is determined. Finally, we have the free variables ''z'' and ''x'', and the variable ''y'' restricted for our choice of the variable ''x''.
in R^3 has the free variable ''z'' and given that we select a value (for example for ''x'') the another value from the variable ''y'' is determined. Finally, we have the free variables ''z'' and ''x'', and the variable ''y'' restricted for our choice of the variable ''x''.
The equation
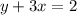 in R^3 (given that we have two free variables) represents a plane.
in R^3 (given that we have two free variables) represents a plane.
Using the same reasoning, the equation
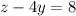 represents a plane (given that it has two free variables : ''y'' and ''x'')
represents a plane (given that it has two free variables : ''y'' and ''x'')
Finally, the pair of equations
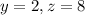 set values for ''y'' and ''z'' leading us ''x'' as the free variable. With only one free variable we will have a ''one dimensional'' geometric form. The one dimensional forms in R^3 are lines.
set values for ''y'' and ''z'' leading us ''x'' as the free variable. With only one free variable we will have a ''one dimensional'' geometric form. The one dimensional forms in R^3 are lines.
The final answer is a) a line.