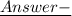
- Given - A trapezium ABCD with non parallel sides of measure 15 cm each ! along , the parallel sides are of measure 13 cm and 25 cm
- To find - Area of trapezium
Refer the figure attached ~
In the given figure ,
AB = 25 cm
BC = AD = 15 cm
CD = 13 cm
Construction -

Now , we can clearly see that AECD is a parallelogram !
 AE = CD = 13 cm
AE = CD = 13 cm
Now ,

Now , In ∆ BCE ,
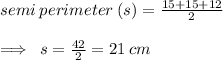
Now , by Heron's formula
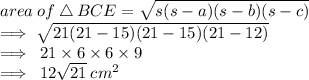
Also ,
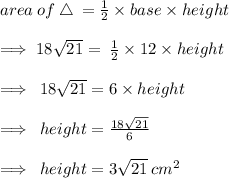
Since we've obtained the height now , we can easily find out the area of trapezium !
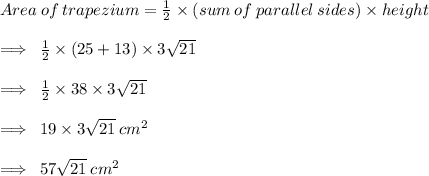
hope helpful :D