Answer:
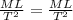
Hence it is proved that Stokes-Oseen formula is dimensionally homogenous.
Step-by-step explanation:
For equation to be dimensionally homogeneous both side of the equation must have same dimensions.
For given Equation:
F= Force, μ= viscosity, D = Diameter, V = velocity, ρ= Density
Dimensions:
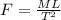
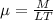
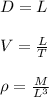
Constants= 1
Now According to equation:
![(ML)/(T^2)=[(M)/(LT)][L] [(L)/(T)] + [(M)/(L^3)][(L^2)/(T^2)][L^2]](https://img.qammunity.org/2021/formulas/engineering/college/gpteol9u73xpb47jk4gta3owidvsibceni.png)
Simplifying above equation, we will get:
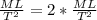
Ignore "2" as it is constant with no dimensions. Now:
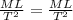
Hence it is proved that Stokes-Oseen formula is dimensionally homogenous.