Question

Use the general slicing method to find the volume of the following solid. The solid whose base is the region bounded by the curve y=38cosx and the​ x-axis on − π 2, π 2​, and whose cross sections through the solid perpendicular to the​ x-axis are isosceles right triangles with a horizontal leg in the​ xy-plane and a vertical leg above the​ x-axis. A coordinate system has an unlabeled x-axis and an unlabeled y-axis. A curve on the x y-plane labeled y equals 38 StartRoot cosine x EndRoot starts on the negative x-axis, rises at a decreasing rate to the positive y-axis, and falls at an increasing rate to the positive x-axis. The region below the curve and above the x-axis is shaded. A right triangle extends from the x y-plane, where one leg is on the x y-plane from the x-axis to the curve and is perpendicular to the x-axis, and the second leg is above the x-axis and is perpendicular to the x y-plane. y=38cosx

Answer 1

To find the volume of the solid described in the question, use the method of slicing. Divide the region bounded by the curve and the x-axis into small slices and find the volume of each slice. Add up the volumes of all the slices to find the total volume of the solid.

Step-by-step explanation:

To find the volume of the solid described in the question, we can use the method of slicing. The cross sections of the solid are isosceles right triangles with one leg along the x-axis and the other leg above the x-axis. We can divide the region bounded by the curve and the x-axis into small slices and find the volume of each slice. The volume of each slice is the area of the triangle (which is equal to 0.5 times the horizontal leg of the triangle) multiplied by the thickness of the slice. By adding up the volumes of all the slices, we can find the total volume of the solid.

Answer 2

The volume of the solid = 1444

Step-by-step explanation:

Given that:

The region of the solid is bounded by the curves
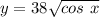`y = 38 √(cos \ x)` and the axis on
`[-(\pi)/(2), (\pi)/(2)]`

using the slicing method

Let say the solid object extends from a to b and the cross-section of the solid perpendicular to the x-axis has an area expressed by function A.

Then, the volume of the solid is ;

`V = \int ^b_a \ A(x) \ dx`

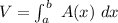
However, each perpendicular slice is an isosceles leg on the xy-plane and vertical leg above the x-axis

Then, the area of the perpendicular slice at a point
`x \ \epsilon \ [-(\pi)/(2),(\pi)/(2)]` is:

`A(x) =(1)/(2) * b * h`

`A(x) =(1)/(2) *(38 √(cos \ x))^2`

`A(x) =(1444)/(2) \ cos \ x`

`A(x) =722 \ cos \ x`

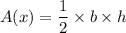
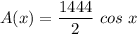
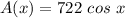
Applying the general slicing method ;

`V = \int ^b_a \ A(x) \ dx \\ \\ V = \int ^{(\pi)/(2) }_{-(\pi)/(2)} (722 \ cos x) \ dx \\ \\ V = 722 \int ^{(\pi)/(2)}_{-(\pi)/(2)} cosx \dx`

`V = 722 [ sin \ x ] ^{(\pi)/(2)}_{-(\pi)/(2)}`

`V = 722 [sin (\pi)/(2) - sin (-(\pi)/(2))]`

`V = 722 [sin (\pi)/(2) + sin (\pi)/(2))]`

`V = 722 [1+1]`

`V = 722 [2]`

V = 1444

∴ The volume of the solid = 1444