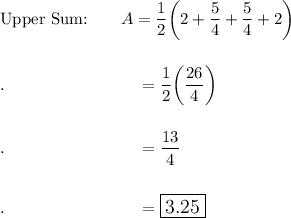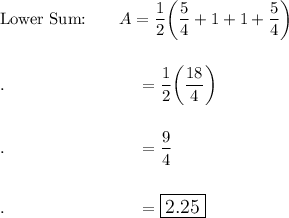Answer: n = 3 n = 4
Upper Sum ≈ 3.41 Upper Sum ≈ 3.25
Lower Sum ≈ 2.15 Lower Sum ≈ 2.25
Explanation:
You are trying to find the area under the curve. Area = height x width.
Height is the y-value at the given coordinate --> f(x)
Width is the distance between the x-values --> dx
n = 3
First, let's figure out dx: the distance from -1 to +1 is 2 units. We need to divide that into 3 sections because n = 3 --> dx = 2/3
So the points we will evaluate is when x = {-1, -1/3, 1/3, 1}
For the upper sum, we find the max y-value for each interval
For the lower sum, we find the min y-value for each interval
Next, let's find the height for each of the x-values:
f(x) = 1 + x²
f(-1) = 1 + (-1)² = 2
f(-1/3) = 1 + (-1/3)² = 1 + 1/9 --> 10/9
f(1/3) = 1 + (1/3)² = 1 + 1/9 --> 10/9
f(1) = 1 + (1)² = 2
Interval Max Min
{-1, -1/3} f(-1) = 2 f(-1/3) = 10/9
{-1/3, 1/3} f(-1/3) = 10/9 f(0) = 1 (vertex lies in this interval)
{1/3, 1} f(1) = 2 f(1/3) = 10/9
Now, let's find the Area: A = f(x) dx:
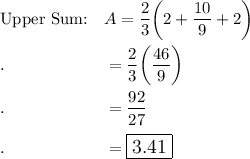
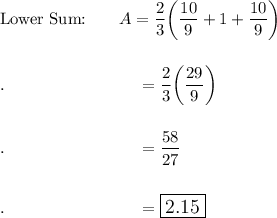
*****************************************************************************************
n = 4
First, let's figure out dx: the distance from -1 to +1 is 2 units. We need to divide that into 4 sections because n = 4 --> dx = 2/4 = 1/2 (simplified)
So the points we will evaluate is when x = {-1, -1/2, 0, 1/2, 1}
For the upper sum, we find the max y-value for each interval
For the lower sum, we find the min y-value for each interval
Next, let's find the height for each of the x-values:
f(x) = 1 + x²
f(-1) = 1 + (-1)² = 2
f(-1/2) = 1 + (-1/2)² = 1 + 1/4 --> 5/4
f(0) = 1 + (0)² = 1
f(1/2) = 1 + (1/2)² = 1 + 1/4 --> 5/4
f(1) = 1 + (1)² = 2
Interval Max Min
{-1, -1/2} f(-1) = 2 f(-1/2) = 5/4
{-1/2, 0} f(-1/2) = 5/4 f(0) = 1
{0, 1/2} f(1/2) = 5/4 f(0) = 1
{1/2, 1} f(1) = 2 f(1/3) = 5/4
Now, let's find the Area: A = f(x) dx: